Hey, remember the before times before hubski and similar when we bought toilet books for 10min bite-sized reads? The book this comes from I still have yellowing away on my downstairs toilet shelf and was one of my faves. Other than doing i squared equals minus 1 instead of going to the most beautiful equation in mathematics, it is a great book. It sits on my shelf between "How to fossilise your hamster." - A New Scientist compilation - and "100 places you will never visit" by Daniel Smith.
Uncle John's Bathroom Reader, News of the Weird, etc. Yeah, that's back when you'd buy a new Guinness Book out of Weekly Reader exactly twice until you realized that you know what? World records don't change that much. Preach. My ODE professor spent like 30 minutes going so nuts while deriving Euler's formula that the professor next door had to come over and tell him to pipe down. I felt like I was staring into The Matrix several years before The Matrix came out. I am buying the shit out of "How to fossilise your hamster" now. That looks like exactly what my going-on-eight-year-old needs.Other than doing i squared equals minus 1 instead of going to the most beautiful equation in mathematics, it is a great book.
Some of the later stuff has been simplified down to its pithy essentials. 14, Schrodinger's Equation, for example. That li'l upside down triangle is doing a lot of work: Not all of it changed things for the better either. 17, Black-Scholes, is just a damn partial differential equation which, in finance world, means it's a fuckin' Elvish incantation from outer Venus because those guys, business majors that they are, think algebra is higher math. More importantly, it's a partial differential equation that basically changed finance in the '80s. Things went worse than expected. Note that "picking up nickels in front of bulldozers" was an epithet hurled at LTCM, the hedge fund that had Myron Scholes on their board, to denigrate their investment strategy as entirely too risky for the yield. Then of course the Fed dumped $4b into it to keep the banking system from collapsing in 1998, establishing a trend. As such, "picking up nickels in front of bulldozers" ceased to be a warning but instead became a sound investment strategy pursued by high frequency traders, options traders, and all those idiots on Reddit trading 3x-inverse VIX.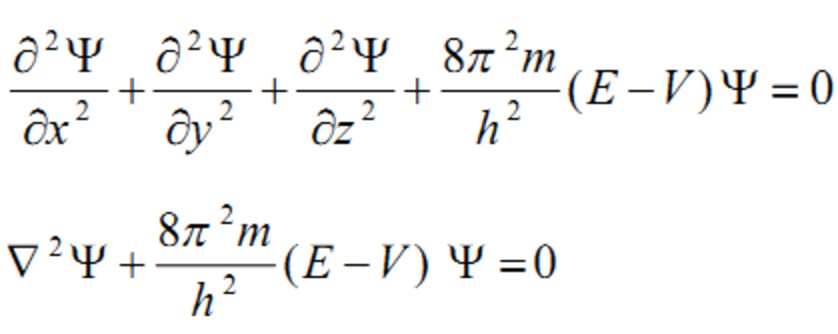

I think I've worked with some form of all of these, except for Euler's polyhedra formula. The only wrong thing I could find is that the lower integration limit on the Fourier transform should be negative infinity. And the Maxwell eqn's given here are for the specific case of in a vacuum. As for Black-Scholes, it's a different form of differential equation than Schrodinger's, so the functions that are solutions to each are quite different.
Both classify as parabolic when considered as time-dependent PDE, so there's a mutual similarity to the heat equation and its solutions.As for Black-Scholes, it's a different form of differential equation than Schrodinger's, so the functions that are solutions to each are quite different.
Thanks for that, makes sense. I must be forgetting why the Schrodinger eq'n is non-dispersive, like why there isn't some first order spatial derivative, similar to the second term of this form of Black-Scholes. I think it's some assumption baked into a particular physical situation. Closed boundary conditions or something, right? It's been too long.